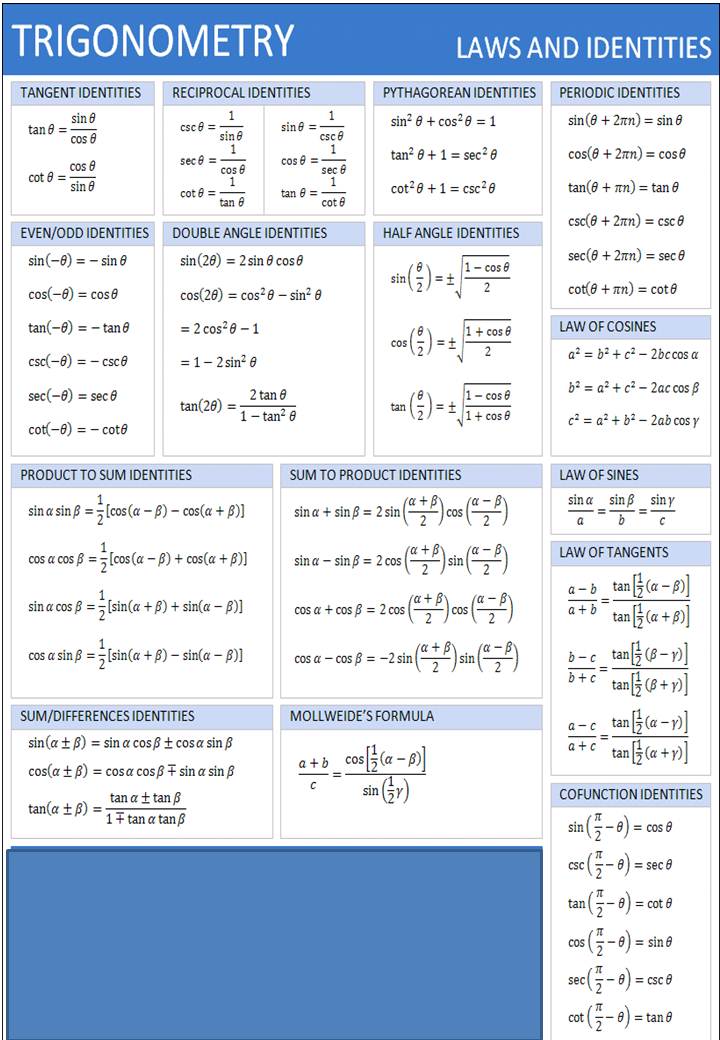
Trigonometry formulas are essential tools in mathematics that help us understand the relationships between the angles and sides of triangles. These formulas are widely used in various fields, including physics, engineering, and architecture. In this article, we will explore the fundamental trigonometric formulas, their applications, and how they can be utilized in solving real-world problems. By the end of this guide, you will have a thorough understanding of trigonometry formulas and their importance in both academic and practical scenarios.

The study of trigonometry dates back to ancient civilizations, where it was primarily used in astronomy and navigation. Today, trigonometry has evolved into a vital branch of mathematics that is instrumental in various scientific and engineering disciplines. Understanding trigonometry formulas is not just about memorizing equations; it is about grasping their applications and implications in real life.

In this article, we will delve into various aspects of trigonometry formulas, including their definitions, types, and practical applications. Whether you are a student preparing for exams or a professional needing to refresh your knowledge, this guide will serve as a valuable resource.

1. Introduction to Trigonometry
2. Basic Trigonometric Formulas
3. Trigonometric Identities
4. Applications of Trigonometry Formulas
5. Advanced Trigonometric Formulas
6. Real-World Examples of Trigonometry
7. Common Errors in Trigonometry
8. Conclusion

1. Introduction to Trigonometry

Trigonometry is a branch of mathematics that studies the relationships between the angles and sides of triangles. The word "trigonometry" is derived from the Greek words "trigonon," meaning triangle, and "metron," meaning measure. The primary focus of trigonometry is on right-angled triangles, where one angle measures 90 degrees.

The fundamental trigonometric ratios are sine (sin), cosine (cos), and tangent (tan), which are defined as follows:

Sine: In a right triangle, the sine of an angle is the ratio of the length of the opposite side to the length of the hypotenuse.
Cosine: The cosine of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse.
Tangent: The tangent of an angle is the ratio of the length of the opposite side to the length of the adjacent side.

2. Basic Trigonometric Formulas

Understanding the basic trigonometric formulas is crucial for solving problems in trigonometry. Here are some of the most important formulas:

2.1 Trigonometric Ratios

The basic trigonometric ratios for a right triangle are:

sin(θ) = Opposite / Hypotenuse
cos(θ) = Adjacent / Hypotenuse
tan(θ) = Opposite / Adjacent

2.2 Pythagorean Identity

One of the fundamental identities in trigonometry is the Pythagorean identity:

sin²(θ) + cos²(θ) = 1

2.3 Angle Sum and Difference Formulas

The angle sum and difference formulas are essential for calculating trigonometric functions of combined angles:

sin(a ± b) = sin(a)cos(b) ± cos(a)sin(b)
cos(a ± b) = cos(a)cos(b) ∓ sin(a)sin(b)
tan(a ± b) = (tan(a) ± tan(b)) / (1 ∓ tan(a)tan(b))

3. Trigonometric Identities

Trigonometric identities are equations that are true for all values of the involved variables. They are essential for simplifying and solving trigonometric equations. Here are some key identities:

3.1 Reciprocal Identities

csc(θ) = 1/sin(θ)
sec(θ) = 1/cos(θ)
cot(θ) = 1/tan(θ)

3.2 Quotient Identities

tan(θ) = sin(θ)/cos(θ)
cot(θ) = cos(θ)/sin(θ)

3.3 Co-Function Identities

sin(90° - θ) = cos(θ)
cos(90° - θ) = sin(θ)
tan(90° - θ) = cot(θ)

4. Applications of Trigonometry Formulas

Trigonometry has a wide range of applications in various fields. Here are some notable applications:

4.1 Engineering

In engineering, trigonometry is used to calculate forces, angles, and distances. Engineers often use trigonometric formulas to design structures and analyze physical systems.

4.2 Astronomy

Astronomers utilize trigonometry to determine distances between celestial bodies and calculate their positions in space.

4.3 Architecture

Architects apply trigonometric principles to create accurate designs, ensuring that buildings are structurally sound and aesthetically pleasing.

5. Advanced Trigonometric Formulas

In addition to basic formulas, there are several advanced trigonometric formulas that are useful for solving complex problems:

5.1 Law of Sines

The law of sines relates the sides of a triangle to the sines of its angles:

a/sin(A) = b/sin(B) = c/sin(C)

5.2 Law of Cosines

The law of cosines is used to find a side of a triangle when the other two sides and the included angle are known:

c² = a² + b² - 2ab*cos(C)

6. Real-World Examples of Trigonometry

To illustrate the practical applications of trigonometry, let’s consider some real-world scenarios:

6.1 Navigation

In navigation, trigonometry is used to calculate distances and bearings between locations. For instance, sailors use trigonometric functions to determine their course when navigating at sea.

6.2 Surveying

Land surveyors employ trigonometry to measure distances and angles when mapping out land. They use the law of sines and cosines to calculate the dimensions of irregular plots.

7. Common Errors in Trigonometry

While working with trigonometry, students often make several common errors. Awareness of these mistakes can help improve understanding:

Confusing the ratios of sine, cosine, and tangent.
Neglecting to simplify trigonometric expressions properly.
Forgetting the unit circle values for specific angles.

8. Conclusion

In conclusion, trigonometry formulas are fundamental tools in mathematics with diverse applications across various fields. Mastering these formulas enables individuals to solve complex problems and understand the world around them better. We encourage you to practice these formulas regularly to solidify your understanding and enhance your mathematical skills.

If you found this article helpful, please leave a comment, share it with others, or check out more articles on our website for further reading